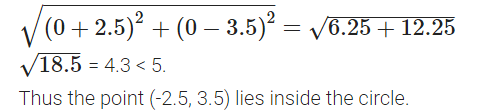Conic Sections (Ex – 11.1)
In each of the following Exercises 1 to 5, find the equation of the circle with
Question 1.
center (0, 2) and radius 2
Solution:
Here h = 0,k = 2 and r = 2
The equation of circle is,
(x-h)2 + (y- k)2 = r2
∴ (x – 0)2 + (y – 2)2 = (2)2
⇒ x2 + y2 + 4 – 4y = 4
⇒ x2 + y2 – 4y = 0
Question 2.
center (-2,3) and radius 4
Solution:
Here h=-2, k = 3 and r = 4
The equation of circle is,
(x – h)2 + (y – k)2 = r2
∴( x + 2)2 + (y – 3)2 = (4)2
⇒ x2 + 4 + 4x + y2 + 9 – 6y = 16
⇒ x2 + y2 + 4x – 6y – 3 = 0
Question 3.
center (1/2,1/4) and radius 1/12
Solution:
here h = 1/2, k = 1/4 and r = 1/12
The equation of circle is,

Question 4.
center (1, 1) and radius √2
Solution:
Here h = l, k=l and r = √2
The equation of circle is,
(x – h)2 + (y – k)2 = r2
∴ (x – 1)2 + (y – 1)2 = (√2)2
⇒ x2 + 1 – 2x + y2 +1 – 2y = 2
⇒ x2 + y2 – 2x – 2y = 0
Question 5.
center (-a, -b) and radius √(a2 − b2).
Solution:
Here h=-a, k = -b and r = √(a2 − b2)
The equation of circle is, (x – h)2 + (y – k)2 = r2
∴ (x + a)2 + (y + b)2 = (√(a2 − b2))
⇒ x2 + a2 + 2ax + y2 + b2 + 2by = a2 -b2
⇒ x2 + y2 + 2ax + 2 by + 2b2 = 0
In each of the following exercises 6 to 9, find the centre and radius of the circles.
Question 6.
(x + 5)2 + (y – 3)2 = 36
Solution:
The given equation of circle is,
(x + 5)2 + (y – 3)2 = 36
⇒ (x + 5)2 + (y – 3)2 = (6)2
Comparing it with (x – h)22 + (y – k)2 = r2, we get
h = -5, k = 3 and r = 6.
Thus the co-ordinates of the center are (-5, 3) and radius is 6.
Question 7.
x2 + y2 – 4x – 8y – 45 = 0
Solution:
The given equation of circle is
x2 + y2 – 4x – 8y – 45 = 0
∴ (x2 – 4x) + (y2 – 8y) = 45
⇒ [x2 – 4x + (2)2] + [y2 – 8y + (4)2] = 45 + (2)2 + (4)2
⇒ (x – 2)2 + (y – 4)2 = 45 + 4 + 16
⇒ (x – 2)2 + (y – 4)2 = 65
⇒ (x – 2)2 + (y – 4)2= (√65)2
Comparing it with (x – h)2 + (y – k)2 = r2, we
have h = 2,k = 4 and r = √65.
Thus co-ordinates of the center are (2, 4) and radius is √65.
Question 8.
x2 + y2 – 8x + 10y – 12 = 0
Solution:
The given equation of circle is,
x2 + y2 – 8x + 10y -12 = 0
∴ (x2 – 8x) + (y2 + 10y) = 12
⇒ [x2 – 8x + (4)2] + [y2 + 10y + (5)2] = 12 + (4)2 + (5)2
⇒ (x – 4)2 + (y + 5)2 = 12 + 16 + 25
⇒ (x – 4)2 + (y + 5)2 = 53
⇒ (x – 4)2 + (y + 5)2 = (53−−√)2
Comparing it with (x – h)2 + (y – k)2 = r2, we have h = 4, k = -5 and r = √53
Thus co-ordinates of the center are (4, -5) and radius is √53.
Question 9.
2x2 + 2y2 – x = 0
Solution:
The given equation of circle is,
2x2 + 2y2 – x = 0

Question 10.
Find the equation of the circle passing through the points (4, 1) and (6, 5) and whose centre is on the line 4x + y = 16.
Solution:
The equation of the circle is,
(x – h)2 + (y – k)2 = r2 ….(i)
Since the circle passes through point (4, 1)
∴ (4 – h)2 + (1 – k)2 = r2
⇒ 16 + h2 – 8h + 1 + k2 – 2k = r2
⇒ h2+ k2 – 8h – 2k + 17 = r2 …. (ii)
Also, the circle passes through point (6, 5)
∴ (6 – h2 + (5 – k)2 = r2
⇒ 36 + h2 -12h + 25 + k2 – 10k = r2
⇒ h2 + k2 – 12h – 10kk + 61 = r2 …. (iii)
From (ii) and (iii), we have h2 + k2 – 8h – 2k +17
= h2 + k2– 12h – 10k + 61
⇒ 4h + 8k = 44 => h + 2k = ll ….(iv)
Since the center (h, k) of the circle lies on the line 4x + y = 16
∴ 4h + k = 16 …(v)
Solving (iv) and (v), we get h = 3 and k = 4.
Putting value of h and k in (ii), we get
(3)2 + (4)2 – 8 x 3 – 2 x 4 + 17 = r2
∴ r2 = 10
Thus required equation of circle is
(x – 3)2 + (y – 4)2 = 10
⇒ x2 + 9 – 6x + y2 +16 – 8y = 10
⇒ x2 + y2 – 6x – 8y +15 = 0.
Question 11.
Find the equation of the circle passing through the points (2, 3) and (-1, 1) and whose center is on the line x – 3y – 11 = 0.
Solution:
The equation of the circle is,
(x – h)2 + (y – k)2 = r2 ….(i)
Since the circle passes through point (2, 3)
∴ (2 – h)2 + (3 – k)2 = r2
⇒ 4 + h2 – 4h + 9 + k2 – 6k = r2
⇒ h2+ k2 – 4h – 6k + 13 = r2 ….(ii)
Also, the circle passes through point (-1, 1)
∴ (-1 – h)2 + (1 – k)2 = r2
⇒ 1 + h2 + 2h + 1 + k2 – 2k = r2
⇒ h2 + k2 + 2h – 2k + 2 = r2 ….(iii)
From (ii) and (iii), we have
h2 + k2 – 4h – 6k + 13 = h2 + k2 + 2h – 2k + 2
⇒ -6h – 4k = -11 ⇒ 6h + 4k = 11 …(iv)
Since the center (h, k) of the circle lies on the line x – 3y-11 = 0.
∴ h – 3k – 11 = 0 ⇒ h -3k = 11 …(v)
Solving (iv) and (v), we get
h = 7/2 and k = −5/2
Putting these values of h and k in (ii), we get
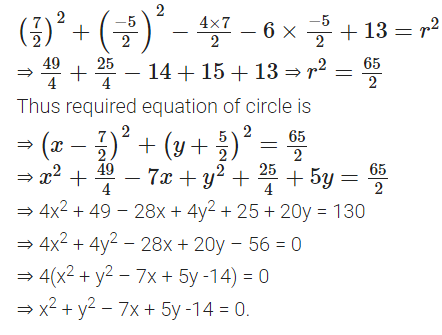
Question 12.
Find the equation of the circle with radius 5 whose centre lies on x-axis and passes through the point (2, 3).
Solution:
Since the center of the circle lies on x-axis, the co-ordinates of center are (h, 0). Now the circle passes through the point (2, 3).
∴ Radius of circle

Question 13.
Find the equation of the circle passing through (0, 0) and making intercepts a and b on the co-ordinate axes.
Solution:
Let the circle makes intercepts a with x-axis and b with y-axis.
∴ OA = a and OB = b
So the co-ordinates of A are (a, 0) and B are (0,b)
Now, the circle passes through three points 0(0, 0), A(a, 0) and B(0, b).

Question 14.
Find the equation of a circle with center (2, 2) and passes through the point (4, 5).
Solution:
The equation of circle is
(x – h)2 + (y – k)2 = r2 ….(i)
Since the circle passes through point (4, 5) and co-ordinates of center are (2, 2)
∴ radius of circle

Question 15.
Does the point (-2.5, 3.5) lie inside, outside or on the circle x2 + y2 = 25?
Solution:
The equation of given circle is x2 + y2 = 25
⇒ (x – 0)2 + (y – 0)2 = (5)2
Comparing it with (x – h)2 + (y – k)2 = r2, we
get
h = 0,k = 0, and r = 5
Now, distance of the point (-2.5, 3.5) from the center (0, 0)