Real Numbers
Question 1.
Prove that √5 is irrational.
Solution:
Let us assume, to the contrary, that √5 is rational.
Squaring on both sides, we get
5 = a2/b2 or 5b2 = a2 ………………. (1)
This shows that a2 is divisible by 5.
It follows that a is divisible by 5. …………………. (2)
So, a = 5m for some integer m.
Substituting a = 5m in (1), we get
5b2 = (5m)2 = 25m2
or b2 = 5m2 or b2 is divisible by 5
and hence b is divisible by 5.
From (2) and (3), we can conclude that 5 is a common factor of both a and b.
But this contradicts our supposition that a and b are coprime.
Hence, √5 is irrational.
Question 2.
Prove that 3 + 2√5 is irrational.
Solution:
Let us assume, to the contrary, that 3 + 2√5 is a rational number.
Now, let 3 + 2√5 = a/b, where a and b are coprime and b ≠ 0.
So, 2√5 = a/b – 3 or √5 = a/2b – 3/2
Since a and b are integers, therefore
a/2b – 3/2 is a rational number.
∴ √5 is an irrational number.
But √5 is an irrational number.
This shows that our assumption is incorrect.
So, 3 + 2√5 is an irrational number.
Question 3.
Prove that the following are irrationals:
- 1/√2
- 7√5
- 6 + √2
Solution:
1. Let us assume, to the contrary, that 1/√2 is rational.
That is, we can find co-prime integers p and q (≠ 0) such that
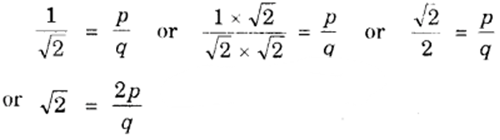
Since p and q are integers, 2p/q is rational, and so √2 is rational.
But this contradicts the fact that √2 is irrational.
So, we conclude that 1/√2 is irrational.
2. Let us assume, to the contrary, that 7√5 is rational.
That is, we can find co-prime integers p and q (≠ 0) such that 7√5 = p/q.
So, √5 = p/7q.
Since p and q are integers, p/7q is rational and so is √5.
But this contradicts the fact that √5 is irrational.
So, we conclude that 7√5 is irrational.
3. Let us assume, to the contrary, that 6 + √2 is rational.
That is, we can find integers p and q (≠ 0) such that
6 + √2 = p/q or 6 – p/q = √2
or √2 = 6 – p/q
Since p and q are integers, we get 6 – p/q is rational, and so √2 is rational.
But this contradicts the fact that √2 is irrational.
So, we conclude that 6 + √2 is irrational.
