Arithmetic Progressions
Question 1.
In which of the following situations, does the list of numbers involved make an arithmetic progression, and why?
(i) The taxi fare after each km when the fare is Rs 15 for the first km and Rs 8 for each additional km.
(ii) The amount of air present in the cylinder when a vacuum pump removes of the air remaining in the cylinder at a time.
(iii) The cost of digging a well after every metre of digging, when it costs Rs 150 for the first metre and rises by Rs 50 for each subsequent metre.
(iv) The amount of money in the account every year, when Rs 10000 is deposited at compound interest at 8% per annum.
Solution:
(i) According to the statement, the fare for journey of 1 km, 2 km, 3 km, 4 km, … are respectively Rs 15, Rs (15 + 8), Rs (15 + 2 × 8), Rs (15 + 3 × 8), …
i.e., 15, 23, 31, 39, …
Since here the terms continually increase by the same number 8, so the list forms an AP.
(ii) Let the amount of air present in the cylinder be x units.
∴ According to the statement, the list giving the air present in the cylinder is given by

∴ These numbers do not form an AP.
(iii) According to the question, the cost of digging a well for the first metre and its succeeding metres in rupees is given by 150, 200, 250, 300, …
Since here the terms continually increase by the same number 50, so the list forms an AP.
(iv) According to the question, the amount of money in the account in the first year and its succeeding years in rupees is given by
10000, 10000(1 + 8/100), 10000(1 + 8/100)2, …………….
i.e; 10000, 10000 × 108/100, 10000 × 108/100 × 108/100, …………..
i.e; 10000, 10800, 11664, ……………
Since 10800 – 10000 ≠ 11664 – 10800, therefore
the list does not form an AP.
Question 2.
Write first four terms of the AP, when the first term a and common difference d are given as follows:
(i) a = 10, d = 10
(ii) a = – 2, d = 0
(iii) a = 4, d = – 3
(iv) a = – 1, d = 1/2
(v) a = – 1.25, d = – 0.25
Solution:
We know that if the first term is a and the common difference is d, then
a, a + d, a + 2d, a + 3d, … represents an AP for different values of a and d.
(i) Putting a = 10 and d = 10 in a, a + d, a + 2d, a + 3d, …, we get the required AP as 10, 10 + 10, 10 + 20, 10 + 30, …….
i.e., 10, 20, 30, 40, …….
(ii) Putting a = – 2 and d = 0 in a, a + d, a + 2d, a + 3d, …, we get the required AP as – 2, – 2 + 0, – 2 + 2 × 0,- 2 + 3 × 0, …
i.e.,- 2, – 2, – 2, – 2, ……….
(iii) Putting a – 4 and d = – 3 in a, a + d, a + 2d, a + 3d, ………….., we get the required AP as 4, 4 – 3, 4 – 6, 4 – 9, …
i.e., 4, 1, – 2, – 5, ……..
(iv) Putting a = – 1 and d = 1/2 in a, a + d, a + 2d, a + 3d, …, we get the required AP as – 1,- 1 + 1/2, – 1 + 2/2, – 1 + 3/2, …………..
i.e; – 1, −1/2, 0, 1/2, ……………
(v) Putting a = – 1.25 and d = – 0.25 in a, a + d, a + 2d, a + 3d, …, we get the required AP as – 1.25, – 1.25 – 0.25, – 1.25 – 0.50, – 1.25 – 0.75, …
i.e; – 1.25, – 1.50, – 1.75, – 2.00, …….
Question 3.
For the following APs, write the first term and the common difference:
(i) 3, 1, – 1, – 3, …
(ii) – 5, – 1, 3, 7, …
(iii) 1/3, 5/3, 9/3, 13/3, …………..
(iv) 0.6, 1.7, 2.8, 3.9 …
Solution:
(i) The given AP is 3, 1, – 1, – 3, …
Clearly, a = 3 and d = 1 – 3 = – 2.
(ii) The given AP is – 5, – 1, 3, 7, …..
Clearly, a = – 5 and d = – 1 – (- 5) = – 1 + 5 = 4
(iii) The given AP is 1/3, 5/3, 9/3, 13/3, ………..
Clearly, a = 1/3 and d = 5/3 – 1/3 = 4/3
(iv) The given AP is 0.6, 1.7, 2.8, 3.9, ………
Clearly, a = 0.6 and d, = 1.7 – 0.6 = 1.1
Question 4.
Which of the following are APs? If they form an AP, find the common difference d and write three more terms.
(i) 2, 4, 8, 16, …….
(ii) 2, 5/2, 3, 7/2, ……………..
(iii) – 1.2, – 3.2, – 5.2, – 7.2, ……….
(iv) – 10, – 6, – 2, 2, ……..
(v) 3, 3 + √2, 3 + 2√2, 3 + 3√2, ………….
(vi) 0.2, 0.22, 0.222, 0.2222, ……….
(vii) 0, – 4, – 8, – 12, …………..
(ix) 1, 3, 9, 27, ……….
(x) a, a2, a3, a4, ……..
(xi) √2, √8, √18, √32, …………..
(xii) √3, √6, √9, √12, …………..
(xiii) √3, √6, √9, √12, …………..
(xiv) 12, 32, 52, 72, …………..
(xv) 12, 52, 72, 73, ………
Solution:
(i) Here, a2 – a1 = 4 – 2 = 2
and a3 – a2 = 8 – 4 = 4
⇒ a2 – a1 ≠ a3 – a2
Thus, 2, 4, 8, 16, ……….. do not form an AP.
(ii) Here, a2 – a1 = 5/2 – 2 = (5−4)/2 = 1/2,
a3 – a2 = 3 – 5/2 = (6−5)/2 = 1/2,
and a4 – a3 = 7/2 – 3 = (7−6)/2 = 1/2
i.e., an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = 1/2
The next three terms after the last given term are:
7/2 + 1/2 = (7+1)/2 = 8/2 = 4,
4 + 1/2 = (8+1)/2 = 9/2,
and 9/2 + 1/2 = (9+1)/2 = 10/2 = 5.
(iii) Here, a2 – a1 = – 3.2 – (- 1.2) = – 3.2 + 1.2 = – 2,
a3 – a2 = – 5.2 – (- 3.2) = – 5.2 + 3.2 = – 2,
and a4 – a3 = – 7.2 – (- 5.2) = – 7.2 + 5.2 = – 2
i.e., an+1 + 1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = – 2
The next three terms after the last given term are:
– 7.2 – 2 = – 9.2,
– 9.2 – 2 = – 11.2,
and – 11.2 – 2 = – 13.2.
(iv) Here, a2 – a1 = – 6 – (- 10) = – 6 + 10 = 4,
a3 – a2 = – 2 – (- 6) = – 2 + 6 = 4,
and a4 – a3 = 2 – (- 2) = 2 + 2 = 4
i.e., an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = 4
The next three terms after the last given term are:
2 + 4 = 6,
6 + 4 = 10, and 10 + 4 = 14.
(v) Here, a2 – a1 = (3 + √2) – 3 = √2
a3 – a2 = (3 + 2√2) – (3 + √2) = √2
and a4 – a3 = (3 + 3√2) – (3 + 2√2) = √2
i.e., a4 – a3 = (3 + 3√2) – (3 + 2√2) = √2
i.e; an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = √2
The next three terms after the last given term are:
(3 + 3√2) + √2 = 3 + 4√2,
(3 + 4√2) + 2–√ = 3 + 52–√,
and (3 + 52–√) + 2–√ = 3 + 62–√.
(vi) Here, a2 – a1 = 0.22 – 0.2 – 0.22 – 0.20 = 0.02
and a3 – a2 = 0.222 – 0.22
= 0.222 – 0.220 = 0.002
∴ a2 – a1 ≠ a3 – a2
Thus, the given list of numbers does not form an AP.
(vii) Here, a2 – a1 = – 4 – 0 = – 4,
a3 – a2 = – 8 – (- 4) = – 8 + 4 = – 4,
and a4 – a3 = – 12 – (- 8) = – 12 + 8 = – 4.
i.e., an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = – 4
The next three terms after the last given term are:
– 12 + (- 4) = – 12 – 4 = – 16,
– 16 + (- 4) = – 16 – 4 = – 20,
and – 20 + (- 4) = – 20 – 4 = – 24.
(viii)
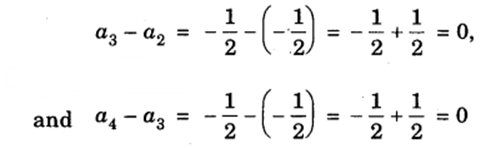
i.e., an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = 0
The next three terms after the last given term are
– 1/2, – 1/2 and – 1/2.
(ix) Here, a2 – a1 = 3 – 1 = 2
and a3 – a2 = 9 – 3 = 6
So, a2 – a1 ≠ a3 – a2
Thus, the given list of numbers does not form an A.P.
(x) Here, a2 – a1 = 2a – a = a,
a3 – a2 = 3a – 2a = a,
and a4 – a3 = 4a – 3a = a
i.e., an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = a
The next three terms after the last given term are:
4a + a = 5a,
5a + a = 6a
and 6a + a = 7a.
(xi) a2 – a1 = a2 – a = a(a – 1)
and a3 – a2 = a3 – a2 = a2(a – 1)
So, a2 – a1 ≠ a3 – a2
Thus, the given list of numbers does not form an AP.
(xii) Here, a2 – a1 = √8 – √2 = √4×2 – √2
= 2√2 – √2 = √2,
a3 – a2 = √18 – √8 = √9×2 – √4×2
= 3√2 – 2√2 = √2
and a4 – a3 = √32 – √18
= √16×2 – √9×2
= 4√2 – 3√2 = √2
i.e; an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = √2
The next three terms after the last given term are

(xiii)
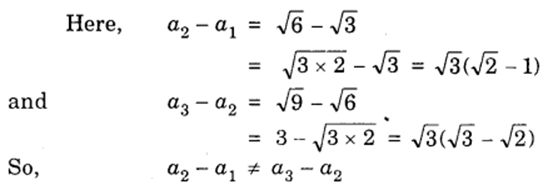
Thus, the given list of numbers does not form an AP.
(xiv) Here, a2 – a1 = 32 – 12 = 9 – 1 = 8,
and a3 – a2 = 52 – 32 = 25 – 9 = 16
So, a2 – a1 ≠ a3 – a2
Thus, the given list of numbers does not form an AP.
(xv) Here, a2 – a1 = 52 – 12 = 25 – 1 = 24,
a3 – a2 = 72 – 52 = 49 – 25 = 24,
and a4 – a3 = 73 – 72 = 73 – 49 = 24
i.e; an+1 – an is same every time. So the given list of numbers forms an AP.
Here, common difference d = 24
The next three terms after the last given term are:
73 + 24 = 97,
97 + 24 = 121,
and 121 + 24 = 145.
